|
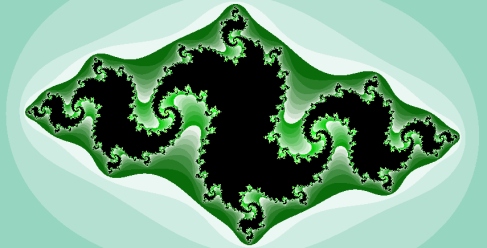
This is an image of the full set, slightly zoomed in, using white for
the interior color rather than the traditional black. This image
also used a non-linear color mapping to bring out the colors a little more. |
 |
This is an image of a classic 8-pointed star, zoomed in about 50x,
near the point -0.415-0.683i. |
 |
This beautiful spiral is shown at a zoom level of about 10000x, but
it goes down into infinity. Spirals like this appear throughout the
edges of the Mandelbrot set. This particular one is near 0.28693186889504513+0.014286693904085048i
with a width of about 6.349e-4. |
 |
Miniature almost-copies of the Mandelbrot Set are spread throughout
the borderlands of the big set. Each copy is at least a little different
than the full set, and each is joined to the full set by an infinite chain
of smaller and smaller additional copies. This specimen was rendered
at a zoom level of about 30,000,000x. |
 |
Whorls and whirlpools appear in many places in the Mandelbrot set,
but to find the nicest ones you have to zoom in. This specimen is
near 0.3245046418497685+0.04855101129280834i at a zoom of about 72 million. |
 |
This is a classic curly Julia set, depicted in 'points' mode.
This Julia set corresponds to seed point 0.3245+0.04855i. |
 |
This is a rather blocky-looking Julia set, selected from a bubble off
the side of the main Mandelbrot Set, at -0.4961+0.5432i. |
 |
This beautiful Julia set was selected from an interior Mandelbrot Set
point very close to the edge, at 0.300283+0.48857i. |
 What is the Mandelbrot Set?
What is the Mandelbrot Set?

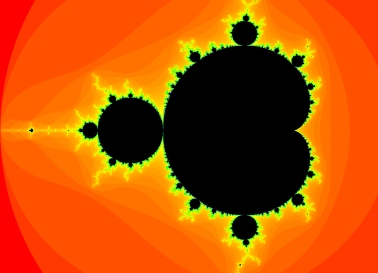
 A Julia set is a set of points in the complex plane, just like a Mandelbrot
set is, but computed with a slightly different approach. Every Julia set
is based on a distinct complex seed value
Cs.
The Julia set for Cs is the set
of values z0 for which magnitude
of |z| in the Mandelbrot formula stays small. So, you can see that
the Mandelbrot Set and Julia sets are related. However, while there is
one unique Mandelbrot Set (for a given power k), there are an infinite
number (technical, at least Aleph1) different Julia sets. The picture at
right shows the Julia set for the point
Cs
= -0.13196-0.81295i.
A Julia set is a set of points in the complex plane, just like a Mandelbrot
set is, but computed with a slightly different approach. Every Julia set
is based on a distinct complex seed value
Cs.
The Julia set for Cs is the set
of values z0 for which magnitude
of |z| in the Mandelbrot formula stays small. So, you can see that
the Mandelbrot Set and Julia sets are related. However, while there is
one unique Mandelbrot Set (for a given power k), there are an infinite
number (technical, at least Aleph1) different Julia sets. The picture at
right shows the Julia set for the point
Cs
= -0.13196-0.81295i.